How to find the range of a data set

Published on September 11, 2020 by Pritha Bhandari. Revised on September 25, 2020.

In statistics, the range is the spread of your data from the lowest to the highest value in the distribution. It is a commonly used measure of variability.

Along with measures of central tendency, measures of variability give you descriptive statistics for summarizing your data set.

The range is calculated by subtracting the lowest value from the highest value. While a large range means high variability, a small range means low variability in a distribution.

Calculate the range

The formula to calculate the range is:

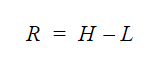
Formula to find the range

  • R = range
  • H = highest value
  • L = lowest value

The range is the easiest measure of variability to calculate. To find the range, follow these steps:

  1. Order all values in your data set from low to high.
  2. Subtract the lowest value from the highest value.

This process is the same regardless of whether your values are positive or negative, or whole numbers or fractions.

Range example
Your data set is the ages of 8 participants.
Participant 1 2 3 4 5 6 7 8
Age 37 19 31 29 21 26 33 36

First, order the values from low to high to identify the lowest value (L) and thehighest value (H).

Age 19 21 26 29 31 33 36 37

Then subtract the lowest from the highest value.

R = H – L

R = 37 – 19 = 18

The range of our data set is 18 years.

How useful is the range?

The range generally gives you a good indicator of variability when you have a distribution without extreme values. When paired with measures of central tendency, the range can tell you about the span of the distribution.

But the range can be misleading when you have outliers in your data set. One extreme value in the data will give you a completely different range.

Range example with an outlier
One value in your data set is replaced with an outlier.
Age 19 21 26 29 31 33 36 61

Using the same calculation, we get a very different result this time:

R = H – L

R = 61 – 19 = 42

With an outlier, our range is now 42 years.

In the example above, the range indicates much more variability in the data than there actually is. Although we have a large range, most values are actually clustered around a clear middle.

Because only two numbers are used, the range is easily influenced by outliers. It can't tell you about the shape of the distribution of values on its own.

To get a clear idea of your data's variability, the range is best used in combination with other measures of variability like interquartile range and standard deviation.

Receive feedback on language, structure and layout

Professional editors proofread and edit your paper by focusing on:

  • Academic style
  • Vague sentences
  • Grammar
  • Style consistency

See an example

Frequently asked questions about the range

Is this article helpful?

You have already voted. Thanks :-) Your vote is saved :-) Processing your vote...